Sort By Grade
Measurement and Data
Geometric measurement: understand concepts of area and relate area to multiplication and to addition.
7Relate area to the operations of multiplication and addition.
bMultiply side lengths to find areas of rectangles with whole-number side lengths in the context of solving real world and mathematical problems, and represent whole-number products as rectangular areas in mathematical reasoning.
3md7b
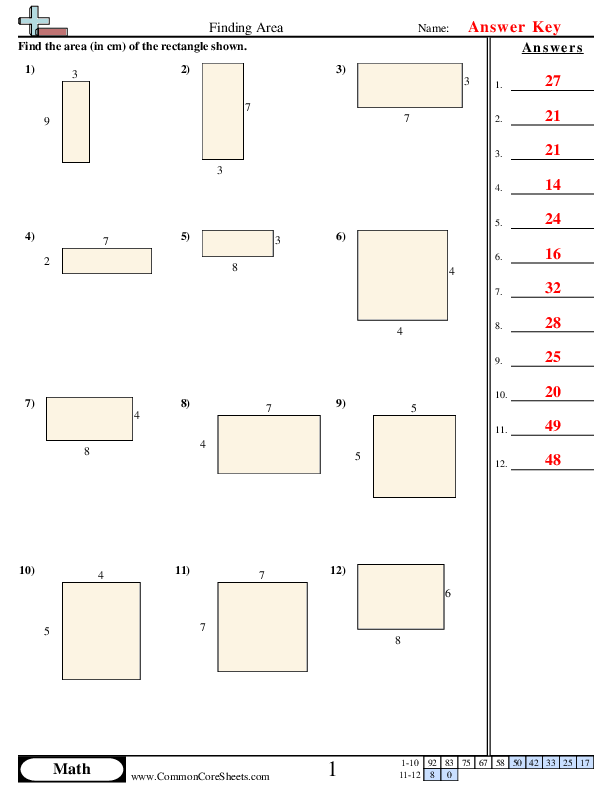
Finding Area
3md7b


×
Description:
"This worksheet is designed to help children grasp the concept of finding areas in mathematics. It presents 12 interactive problems, each featuring a unique geometric figure with set dimensions. Kids can customize the worksheet, transforming it into flash cards to encourage self-directed learning and facilitate memory retention. It's also optimized for use in distance learning scenarios, making it a versatile tool for modern education."

×
Student Goals:
Master the Concept of AreaUpon completion of this worksheet, students should have a sound understanding of the concept of area. They should be able to define area as a measure of the space within a shape and distinguish it from other geometrical attributes like perimeter or volume.Apply Area CalculationsStudents should be able to calculate the area of a rectangle or a square using the formula for the same, i.e., length times width. They should also grasp that these fundamental calculations form the base for determining the size of more complex shapes.Enhance Multiplication SkillsThe regular practice of these area problems should strengthen students' multiplication skills. Students should become proficient in quickly and accurately multiplying different sets of numbers.Develop Problem-Solving SkillsHaving solved a dedicated set of varied problems, students should have boosted their problem-solving capabilities. They should be skilled in critically understanding a problem, breaking it down into simpler tasks and resolving it methodically.Improve Mathematical FluencyThe worksheet can boost mathematical fluency, given its repetitive and progressive structure. Students should be confident in dealing with similar problems and should be adept at applying the learnt area formula over different sets of problems.Promote Real-World ConnectionsWorking through these area problems, students should be able to understand the applications of calculating area in real-world situations. This knowledge enables them to create a strong link between the abstract mathematical world and their daily life.Enhance Analytical ThinkingLastly, the exercise should heighten students' analytical thinking capabilities. They should have a comfortable understanding of the principles behind the math. Furthermore, they should be able to understand patterns, make mathematical connections, and recognize how a change in one dimension (length or width) influences the final area.


